Research Highlights
Researchers uncover topologically protected edge modes in open quantum systems
The authors have demonstrated that topologically protected gapless edge modes can persist when a quantum system is coupled to an external environment.
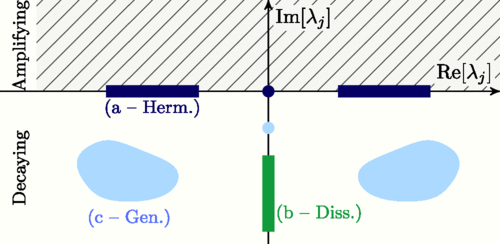
Complex spectra of one-dimensional examples of [(a), dark blue] closed Hermitian systems; [(b), green] purely dissipative systems; and [(c), light blue] generic quadratic Lindbladians studied in this work. Hermitian systems in a topological phase possess in-gap states with zero eigenvalue (dark blue dot), however the topology of purely dissipative systems is not reflected in the Lindblad spectrum. On the other hand, a quadratic Lindbladian which is gapped in the real direction can possess robust zero-frequency edge modes (light blue dot).
It is well known that isolated quantum systems can possess these intriguing edge excitations, but much less is known about their fate in open systems, where the system is coupled to an external environment. Here it is shown that these modes do still persist in the open regime, remaining gapless in energy (phase oscillation frequency), while acquiring a non-zero damping rate. Focussing on systems of non-interacting fermions coupled to memoryless environments, the authors determine a set of symmetry requirements that the open system must obey to prevent the destruction of edge modes, compatible with the constraint that dissipation can induce damping, but not amplification. However, in contrast to closed systems, where the presence of gapless excitations is due to topological properties of the ground state, the authors show that edge modes in an open system can persist when the steady state is trivial. This work provides a general framework to understand symmetry-protected topological phases in the presence of dissipation from an external environment, and uncovers universal features in the non-equilibrium approach of open quantum systems towards their steady states.
Tenfold Way for Quadratic Lindbladians S Lieu, M McGinley and NR Cooper, Phys. Rev. Lett. 124 040401 (2020)
See also the highlight in Nature Physics