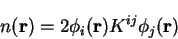Next: Total energy
Up: Density-kernel derivatives
Previous: Kinetic and pseudopotential energies
Contents
The sum of the Hartree and exchange-correlation energies,
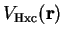 depends only on the density so that
depends only on the density so that
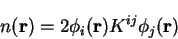 |
(7.23) |
The functional derivative of the Hartree-exchange-correlation energy with
respect to the electronic density is simply the sum of the Hartree and
exchange-correlation potentials,
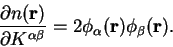 . The
electronic density is given in terms of the density-kernel by
. The
electronic density is given in terms of the density-kernel by
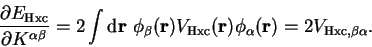 |
(7.24) |
so that we obtain
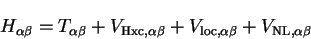 |
(7.25) |
Finally, therefore
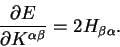 |
(7.26) |
Peter D. Haynes
1999-09-21
![]() depends only on the density so that
depends only on the density so that