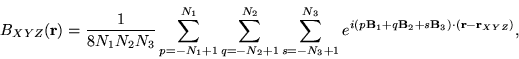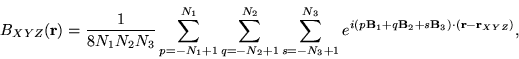Next: Bibliography
Up: Basis Set
Previous: Analytic Integrals
In addition, we define a set of fine grid basis functions with twice the cut-off frequency as the  :
:
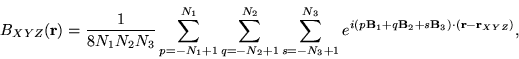 |
(53) |
that correspond to a plane-wave representation that has twice the wavevector cutoff of the standard basis functions.
 are the points of the fine grid. This basis is localised on the grid points of the fine grid and is also orthogonal:
are the points of the fine grid. This basis is localised on the grid points of the fine grid and is also orthogonal:
and satisfies a property analogous to equation (52):
where
 is a version of the function
is a version of the function  that is bandwidth limited to the same frequencies as the basis functions
that is bandwidth limited to the same frequencies as the basis functions  .
.



Next: Bibliography
Up: Basis Set
Previous: Analytic Integrals
Peter D. Haynes
2002-10-29