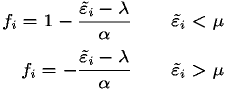

Next: Results
Up: Contents
Previous: Purifying transformation
Condition (1) requires that the coefficients
 depend upon the Hamiltonian eigenvalues
depend upon the Hamiltonian eigenvalues
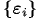 .
Resulting condition number
.
Resulting condition number 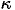 of the Hessian of
of the Hessian of
![$Q[\rho]$](img24.png) is
is![[*]](footnote.png)
Instead start by imposing condition (2), making the
 independent of state
independent of state  (so that
(so that
 ) e.g.
) e.g.
For
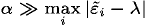 , the minimum occurs for
, the minimum occurs for
i.e. for approximately idempotent density-matrices.
Peter D. Haynes
2001-11-19
![[*]](footnote.png)